Actions




Next: Actions
Up: Algebraic Combinatorics Via Finite
Previous: Contents
This introductory chapter contains the basic notions:
actions of groups,
orbits,
stabilizers,
fixed points,
and so on.
Various examples are given. The
Cauchy-Frobenius Lemma
is derived,
which yields the number of orbits in the case when both
the group and the set on which it acts are finite.
In order
to prepare the applications of this lemma and its refinements which follow
in the next chapters, a detailed description of the
conjugacy classes of
symmetric
and of
monomial groups is added.
The
paradigmatic actions
which
we discuss and apply in full detail here and later on are several natural
actions on the set  ,
consisting of all the mappings from
,
consisting of all the mappings from  into
into 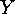 .
These actions on
.
These actions on  are induced in a natural way
by actions of groups on
are induced in a natural way
by actions of groups on  or on
or on 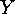 .
The corresponding orbits are called symmetry classes of
mappings and there are many structures in mathematics and sciences which
can be defined as symmetry classes of this kind.
.
The corresponding orbits are called symmetry classes of
mappings and there are many structures in mathematics and sciences which
can be defined as symmetry classes of this kind.
The
enumeration of symmetry classes of mappings
is described in full detail,
in order to prepare refinements which are given in the following chapters.
A very simple case of a group action leads to another important enumerative
concept,
the so-called
involution principle.
Finally we discuss the enumeration of
symmetry classes which consist of
injective or
surjective mappings only.
Herr Fripertinger
Sun Feb 05 18:28:26 MET 1995
 ,
consisting of all the mappings from
,
consisting of all the mappings from  into
into 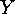 .
These actions on
.
These actions on  are induced in a natural way
by actions of groups on
are induced in a natural way
by actions of groups on  or on
or on 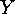 .
The corresponding orbits are called symmetry classes of
mappings and there are many structures in mathematics and sciences which
can be defined as symmetry classes of this kind.
.
The corresponding orbits are called symmetry classes of
mappings and there are many structures in mathematics and sciences which
can be defined as symmetry classes of this kind.