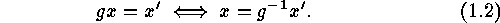
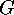 on
on  has first of all the following property which is
immediate from the two conditions mentioned in its definition:
has first of all the following property which is
immediate from the two conditions mentioned in its definition:
This is the reason for the fact that 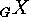 induces several structures on
induces several structures on  and
and 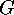 , and
it is the close arithmetic and algebraic connection between these
structures which makes the concept of group action so efficient.
First of all the action induces the following equivalence
relation on
, and
it is the close arithmetic and algebraic connection between these
structures which makes the concept of group action so efficient.
First of all the action induces the following equivalence
relation on  (exercise
(exercise ![]() ):
):
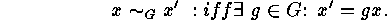
The equivalence classes are called orbits , and
the orbit of 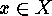 will be indicated as follows:
will be indicated as follows:
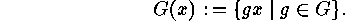
As 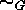 is an equivalence relation on
is an equivalence relation on  , a transversal
, a transversal
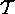 of the orbits yields a set partition
of
of the orbits yields a set partition
of  , i.e. a complete
dissection of
, i.e. a complete
dissection of  into the pairwise disjoint and
nonempty subsets
into the pairwise disjoint and
nonempty subsets 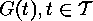 :
:
The set of orbits will be denoted by
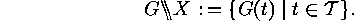
In the case when both 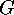 and
and  are finite, we call the action a
finite action .
We notice that, according to
are finite, we call the action a
finite action .
We notice that, according to ![]() , for each
finite
, for each
finite 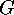 -set
-set  , we may also assume without loss of generality
that
, we may also assume without loss of generality
that 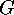 is finite.
If G has exactly one orbit on
is finite.
If G has exactly one orbit on  , i.e. if and only if
, i.e. if and only if
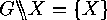 , then we say that the action is transitive,
or
that
, then we say that the action is transitive,
or
that 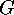 acts transitively on
acts transitively on  .
.
According to ![]() an action of
an action of 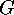 on
on  yields a
partition of
yields a
partition of  . It is trivial but very important
to notice that also the converse is true: Each
set partition of
. It is trivial but very important
to notice that also the converse is true: Each
set partition of  gives rise to an action of a certain group
gives rise to an action of a certain group 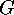 on
on  as follows. Let, for an index set
as follows. Let, for an index set 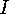 ,
, 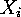 ,
, 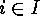 , denote the
blocks of the set partition in question, i.e. the
, denote the
blocks of the set partition in question, i.e. the 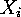 are nonempty, pairwise
disjoint, and their union is equal to
are nonempty, pairwise
disjoint, and their union is equal to  . Then the following subgroup of the
symmetric group
. Then the following subgroup of the
symmetric group 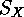 acts in a natural way on
acts in a natural way on  and has the
and has the 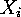 as its
orbits:
as its
orbits:
Summarizing our considerations in two sentences, we have obtained:
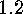 .
. Corollary
An action of a group
Corollary
An action of a group 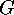 on a set
on a set  is equivalent to a permutation
representation of
is equivalent to a permutation
representation of 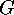 on
on  and it yields a set partition of
and it yields a set partition of  into
orbits. Conversely, each set partition of
into
orbits. Conversely, each set partition of  corresponds in a natural
way to an action of a certain subgroup of the symmetric group
corresponds in a natural
way to an action of a certain subgroup of the symmetric group 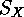 which has
the blocks of the partition as its orbits.
which has
the blocks of the partition as its orbits.
Exercises
E  .
. Prove that
Prove that 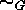 is in fact an equivalence relation.
is in fact an equivalence relation.